Dinamički sistem

Lorencov atraktor se javlja u studiji Lorencovog oscilatora, koji je dinamički sistem.

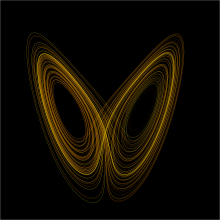
U matematici, dinamički sistem je sistem u kome funkcija opisuje vremensku zavisnost od tačke u geometrijskom prostoru. Primeri obuhvataju matematičke modele koji opisuju njihanje klatna časovnika, protok vode u cevi, i broj riba svakog proleća u jezeru.

At any given time, a dynamical system has a state given by a tuple of real numbers (a vector) that can be represented by a point in an appropriate state space (a geometrical manifold). The evolution rule of the dynamical system is a function that describes what future states follow from the current state. Often the function is deterministic, that is, for a given time interval only one future state follows from the current state. However, some systems are stochastic, in that random events also affect the evolution of the state variables.

In physics, a dynamical system is described as a "particle or ensemble of particles whose state varies over time and thus obeys differential equations involving time derivatives." In order to make a prediction about the system’s future behavior, an analytical solution of such equations or their integration over time through computer simulation is realized.

The study of dynamical systems is the focus of dynamical systems theory, which has applications to a wide variety of fields such as mathematics, physics, biology, chemistry, engineering, economics, and medicine. Dynamical systems are a fundamental part of chaos theory, logistic map dynamics, bifurcation theory, the self-assembly and self-organization processes, and the edge of chaos concept.

Pregled

The concept of a dynamical system has its origins in Newtonian mechanics. There, as in other natural sciences and engineering disciplines, the evolution rule of dynamical systems is an implicit relation that gives the state of the system for only a short time into the future. (The relation is either a differential equation, difference equation or other time scale.) To determine the state for all future times requires iterating the relation many times—each advancing time a small step. The iteration procedure is referred to as solving the system or integrating the system. If the system can be solved, given an initial point it is possible to determine all its future positions, a collection of points known as a trajectory or orbit.

Before the advent of computers, finding an orbit required sophisticated mathematical techniques and could be accomplished only for a small class of dynamical systems. Numerical methods implemented on electronic computing machines have simplified the task of determining the orbits of a dynamical system.

For simple dynamical systems, knowing the trajectory is often sufficient, but most dynamical systems are too complicated to be understood in terms of individual trajectories. The difficulties arise because:

• The systems studied may only be known approximately—the parameters of the system may not be known precisely or terms may be missing from the equations. The approximations used bring into question the validity or relevance of numerical solutions. To address these questions several notions of stability have been introduced in the study of dynamical systems, such as Lyapunov stability or structural stability. The stability of the dynamical system implies that there is a class of models or initial conditions for which the trajectories would be equivalent. The operation for comparing orbits to establish their equivalence changes with the different notions of stability.
• The type of trajectory may be more important than one particular trajectory. Some trajectories may be periodic, whereas others may wander through many different states of the system. Applications often require enumerating these classes or maintaining the system within one class. Classifying all possible trajectories has led to the qualitative study of dynamical systems, that is, properties that do not change under coordinate changes. Linear dynamical systems and systems that have two numbers describing a state are examples of dynamical systems where the possible classes of orbits are understood.
• The behavior of trajectories as a function of a parameter may be what is needed for an application. As a parameter is varied, the dynamical systems may have bifurcation points where the qualitative behavior of the dynamical system changes. For example, it may go from having only periodic motions to apparently erratic behavior, as in the transition to turbulence of a fluid.
• The trajectories of the system may appear erratic, as if random. In these cases it may be necessary to compute averages using one very long trajectory or many different trajectories. The averages are well defined for ergodic systems and a more detailed understanding has been worked out for hyperbolic systems. Understanding the probabilistic aspects of dynamical systems has helped establish the foundations of statistical mechanics and of chaos.

Literatura

• Ralph Abraham; Jerrold E. Marsden (1978). Foundations of mechanics. Benjamin–Cummings. ISBN 978-0-8053-0102-1.  (available as a reprint: ISBN 0-201-40840-6)
• Encyclopaedia of Mathematical Sciences (ISSN 0938-0396) has a sub-series on dynamical systems with reviews of current research.
• Christian Bonatti; Lorenzo J. Díaz; Marcelo Viana (2005). Dynamics Beyond Uniform Hyperbolicity: A Global Geometric and Probabilistic Perspective. Springer. ISBN 978-3-540-22066-4. 
• Stephen Smale (1967). „Differentiable dynamical systems”. Bulletin of the American Mathematical Society. 73 (6): 747—817. doi:10.1090/S0002-9904-1967-11798-1. 
• V. I. Arnold (1982). Mathematical methods of classical mechanics. Springer-Verlag. ISBN 978-0-387-96890-2. 
• Jacob Palis; Welington de Melo (1982). Geometric theory of dynamical systems: an introduction. Springer-Verlag. ISBN 978-0-387-90668-3. 
• David Ruelle (1989). Elements of Differentiable Dynamics and Bifurcation Theory. Academic Press. ISBN 978-0-12-601710-6. 
• Tim Bedford; Michael Keane; Caroline Series, ур. (1991). Ergodic theory, symbolic dynamics and hyperbolic spaces. Oxford University Press. ISBN 978-0-19-853390-0. 
• Ralph H. Abraham; Christopher D. Shaw (1992). Dynamics—the geometry of behavior, 2nd edition. Addison-Wesley. ISBN 978-0-201-56716-8. 
• Kathleen T. Alligood; Tim D. Sauer; James A. Yorke (2000). Chaos. An introduction to dynamical systems. Springer Verlag. ISBN 978-0-387-94677-1. 
• Oded Galor (2011). Discrete Dynamical Systems. Springer. ISBN 978-3-642-07185-0. 
• Morris W. Hirsch; Stephen Smale; Robert L. Devaney (2003). Differential Equations, dynamical systems, and an introduction to chaos. Academic Press. ISBN 978-0-12-349703-1. 
• Anatole Katok; Boris Hasselblatt (1996). Introduction to the modern theory of dynamical systems. Cambridge. ISBN 978-0-521-57557-7. 
• Stephen Lynch (2010). Dynamical Systems with Applications using Maple 2nd Ed. Springer. ISBN 978-0-8176-4389-8. 
• Stephen Lynch (2014). Dynamical Systems with Applications using MATLAB 2nd Edition. Springer International Publishing. ISBN 978-3319068190. 
• Stephen Lynch (2017). Dynamical Systems with Applications using Mathematica 2nd Ed. Springer. ISBN 978-3-319-61485-4. 
• Stephen Lynch (2018). Dynamical Systems with Applications using Python. Springer International Publishing. ISBN 978-3-319-78145-7. 
• James Meiss (2007). Differential Dynamical Systems. SIAM. ISBN 978-0-89871-635-1. 
• David D. Nolte (2015). Introduction to Modern Dynamics: Chaos, Networks, Space and Time. Oxford University Press. ISBN 978-0199657032. 
• Julien Clinton Sprott (2003). Chaos and time-series analysis. Oxford University Press. ISBN 978-0-19-850839-7. 
• Steven H. Strogatz (1994). Nonlinear dynamics and chaos: with applications to physics, biology chemistry and engineering. Addison Wesley. ISBN 978-0-201-54344-5. 
• Teschl, Gerald (2012). Ordinary Differential Equations and Dynamical Systems. Providence: American Mathematical Society. ISBN 978-0-8218-8328-0. 
• Stephen Wiggins (2003). Introduction to Applied Dynamical Systems and Chaos. Springer. ISBN 978-0-387-00177-7. 
• Florin Diacu; Philip Holmes (1996). Celestial Encounters. Princeton. ISBN 978-0-691-02743-2. 
• James Gleick (1988). Chaos: Making a New Science. Penguin. ISBN 978-0-14-009250-9. 
• Ivar Ekeland (1990). Mathematics and the Unexpected (Paperback). University Of Chicago Press. ISBN 978-0-226-19990-0. 
• Ian Stewart (1997). Does God Play Dice? The New Mathematics of Chaos. Penguin. ISBN 978-0-14-025602-4. 

Spoljašnje veze

• Arxiv preprint server has daily submissions of (non-refereed) manuscripts in dynamical systems.
• Encyclopedia of dynamical systems A part of Scholarpedia — peer reviewed and written by invited experts.
• Nonlinear Dynamics. Models of bifurcation and chaos by Elmer G. Wiens
• Sci.Nonlinear FAQ 2.0 (Sept 2003) provides definitions, explanations and resources related to nonlinear science

Onlajn knjige i beleške sa predavanja

• Geometrical theory of dynamical systems. Nils Berglund's lecture notes for a course at ETH at the advanced undergraduate level.
• Dynamical systems. George D. Birkhoff's 1927 book already takes a modern approach to dynamical systems.
• Chaos: classical and quantum. An introduction to dynamical systems from the periodic orbit point of view.
• Learning Dynamical Systems. Tutorial on learning dynamical systems.
• Ordinary Differential Equations and Dynamical Systems. Lecture notes by Gerald Teschl

Istraživačke grupe

• Dynamical Systems Group Groningen, IWI, University of Groningen.
• Chaos @ UMD. Concentrates on the applications of dynamical systems.
• [1], SUNY Stony Brook. Lists of conferences, researchers, and some open problems.
• Center for Dynamics and Geometry, Penn State.
• Control and Dynamical Systems, Caltech.
• Laboratory of Nonlinear Systems, Ecole Polytechnique Fédérale de Lausanne (EPFL).
• Center for Dynamical Systems, University of Bremen
• Systems Analysis, Modelling and Prediction Group, University of Oxford
• Non-Linear Dynamics Group, Instituto Superior Técnico, Technical University of Lisbon
• Dynamical Systems, IMPA, Instituto Nacional de Matemática Pura e Applicada.
• Nonlinear Dynamics Workgroup, Institute of Computer Science, Czech Academy of Sciences.
• UPC Dynamical Systems Group Barcelona, Polytechnical University of Catalonia.
• Center for Control, Dynamical Systems, and Computation, University of California, Santa Barbara.