Kompaktan prostor

Interval A = (−∞, −2] nije kompaktan zato što nije ograničen. Interval C = (2, 4) nije kompktan zato što nije zatvoren. Interval B = [0, 1] je kompaktan zato što je zatvoren i ograničen.

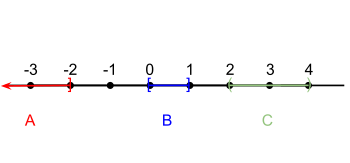
U matematici, i specifičnije opštoj topologiji, kompaktnost je svojstvo koje generalizuje pojam podskupa Euklidovog prostora koji je zatvoren (da sadrži sve svoje granične tačke) i ograničen (onaj kod koga sve njegove tačke leže na datom fiksnom rastojanju jedna od druge). Primeri su zatvoreni interval, četvorougao, ili konačni set tačaka. Ovaj je pojam definisan za opštije topološke prostore, nego što je Euklidov prostor na razne načine.

Jedna takva generalizacija je da je topološki prostor sekvencijalno kompaktan ako svaki infinitivni niz tačaka uzet kao uzorak prostora ima beskonačni podniz koji konvergira u istu tačku prostora. Bolcano-Vajerštrasova teorema navodi da je podskup Euklidovog prostora kompaktan u ovom sekvencijalnom smislu ako i samo ako je zatvoren i ograničen. Stoga, ako se izabere beskonačan broj tačaka u zatvorenom jediničnom intervalu [0, 1] neke od tih tačaka će biti proizvoljno blizo nekim realnom broju u tom prostoru. Na primer, neki od brojeva 1/2, 4/5, 1/3, 5/6, 1/4, 6/7, … se akumuliraju do 0 (drugi se akumuliraju do 1). Isti skup tačaka se ne bi akumulirao do bilo koje tačke otvorenog jediničnog intervala (0, 1); tako da otvoreni jedinični interval nije kompaktan. Sam Euklidov prostor nije kompaktan, jer nije ograničen. Na primer, niz tačaka 0, 1, 2, 3, … nije niz koji konvergira u bilo koji realni broj.

Osim zatvorenih i ograničenih podskupova Euklidovog prostora, tipični primeri kompaktnih prostora obuhvataju prostore koji se ne sastoje od geometrijskih tačaka već od funkcija. Termin kompaktan je uveo u matematiku Moris Freše 1904. godine kao destilaciju ovog koncepta. Kompaktnost u ovoj generalnijoj situaciji igra ekstremno važnu ulogu u matematičkoj analizi, zato što se mnoge klasične i važne teoreme analize 19. veka, kao što je teorema ekstremne vrednosti, lako generalizuju u ovoj situaciji. Tipičnu primenu pruža Arcela-Askolijeva teorema ili Peanova teorema postojanja, prema kojoj je moguće izvesti zaključak o postojanju funkcije s nekim traženim svojstvima kao ograničavajući slučaj date elementarnije konstrukcije. Nakon njegovog početnog uvođenja, različiti ekvivalentni pojmovi kompaktnosti, uključujući sekvencijalnu kompaktnost i kompaktnost granične tačke, razvijeni su u opštim metričkim prostorima.

Istorijski razvoj

In the 19th century, several disparate mathematical properties were understood that would later be seen as consequences of compactness. On the one hand, Bernard Bolzano (1817) had been aware that any bounded sequence of points (in the line or plane, for instance) has a subsequence that must eventually get arbitrarily close to some other point, called a limit point. Bolzano's proof relied on the method of bisection: the sequence was placed into an interval that was then divided into two equal parts, and a part containing infinitely many terms of the sequence was selected. The process could then be repeated by dividing the resulting smaller interval into smaller and smaller parts — until it closes down on the desired limit point. The full significance of Bolzano's theorem, and its method of proof, would not emerge until almost 50 years later when it was rediscovered by Karl Weierstrass.

In the 1880s, it became clear that results similar to the Bolzano–Weierstrass theorem could be formulated for spaces of functions rather than just numbers or geometrical points. The idea of regarding functions as themselves points of a generalized space dates back to the investigations of Giulio Ascoli and Cesare Arzelà. The culmination of their investigations, the Arzelà–Ascoli theorem, was a generalization of the Bolzano–Weierstrass theorem to families of continuous functions, the precise conclusion of which was that it was possible to extract a uniformly convergent sequence of functions from a suitable family of functions. The uniform limit of this sequence then played precisely the same role as Bolzano's "limit point". Towards the beginning of the twentieth century, results similar to that of Arzelà and Ascoli began to accumulate in the area of integral equations, as investigated by David Hilbert and Erhard Schmidt. For a certain class of Green's functions coming from solutions of integral equations, Schmidt had shown that a property analogous to the Arzelà–Ascoli theorem held in the sense of mean convergence — or convergence in what would later be dubbed a Hilbert space. This ultimately led to the notion of a compact operator as an offshoot of the general notion of a compact space. It was Maurice Fréchet who, in 1906, had distilled the essence of the Bolzano–Weierstrass property and coined the term compactness to refer to this general phenomenon (he used the term already in his 1904 paper which led to the famous 1906 thesis).

Literatura

• Alexandrov, Pavel; Urysohn, Pavel (1929), „Mémoire sur les espaces topologiques compacts”, Koninklijke Nederlandse Akademie van Wetenschappen te Amsterdam, Proceedings of the section of mathematical sciences, 14 .
• Arkhangel'skii, A.V.; Fedorchuk, V.V. (1990), „The basic concepts and constructions of general topology”, Ур.: Arkhangel'skii, A.V.; Pontrjagin, L.S., General topology I, Encyclopedia of the Mathematical Sciences, 17, Springer, ISBN 978-0-387-18178-3 .
• Arkhangel'skii, A.V. (2001). „Compact space”. Ур.: Hazewinkel Michiel. Encyclopaedia of Mathematics. Springer. ISBN 978-1556080104. .
• Bolzano, Bernard (1817), Rein analytischer Beweis des Lehrsatzes, dass zwischen je zwey Werthen, die ein entgegengesetzes Resultat gewähren, wenigstens eine reele Wurzel der Gleichung liege, Wilhelm Engelmann  (Purely analytic proof of the theorem that between any two values which give results of opposite sign, there lies at least one real root of the equation).
• Borel, Émile (1895), „Sur quelques points de la théorie des fonctions”, Annales Scientifiques de l'École Normale Supérieure, 3, 12: 9—55, JFM 26.0429.03 
• Boyer, Carl B. (1959), The history of the calculus and its conceptual development, New York: Dover Publications, MR 0124178 .
• Arzelà, Cesare (1895), „Sulle funzioni di linee”, Mem. Accad. Sci. Ist. Bologna Cl. Sci. Fis. Mat., 5 (5): 55—74 .
• Arzelà, Cesare (1882—1883), „Un'osservazione intorno alle serie di funzioni”, Rend. Dell' Accad. R. Delle Sci. Dell'Istituto di Bologna: 142—159 .
• Ascoli, G. (1883—1884), „Le curve limiti di una varietà data di curve”, Atti della R. Accad. Dei Lincei Memorie della Cl. Sci. Fis. Mat. Nat., 18 (3): 521—586 .
• Fréchet, Maurice (1906), „Sur quelques points du calcul fonctionnel”, Rendiconti del Circolo Matematico di Palermo, 22 (1): 1—72, doi:10.1007/BF03018603 .
• Gillman, Leonard; Jerison, Meyer (1976), Rings of continuous functions, Springer-Verlag .
• Kelley, John (1955), General topology, Graduate Texts in Mathematics, 27, Springer-Verlag .
• Kline, Morris (1972), Mathematical thought from ancient to modern times (3rd изд.), Oxford University Press (објављено 1990), ISBN 978-0-19-506136-9 .
• Lebesgue, Henri (1904), Leçons sur l'intégration et la recherche des fonctions primitives, Gauthier-Villars .
• Robinson, Abraham (1996), Non-standard analysis, Princeton University Press, ISBN 978-0-691-04490-3, MR 0205854 .
• Scarborough, C.T.; Stone, A.H. (1966), „Products of nearly compact spaces” (PDF), Transactions of the American Mathematical Society, Transactions of the American Mathematical Society, Vol. 124, No. 1, 124 (1): 131—147, JSTOR 1994440, doi:10.2307/1994440 .
• Steen, Lynn Arthur; Seebach, J. Arthur Jr. (1995) [1978], Counterexamples in Topology (Dover Publications reprint of 1978 изд.), Berlin, New York: Springer-Verlag, ISBN 978-0-486-68735-3, MR 507446 
• Willard, Stephen (1970), General Topology, Dover publications, ISBN 978-0-486-43479-7 
• Adams, Robert A. (1995). Calculus : A Complete Course. Reading: Addison-Wesley. стр. 706—707. ISBN 0-201-82823-5. 
• Rudin, Walter (1976). Principles of Mathematical Analysis. New York: McGraw Hill. стр. 89—90. ISBN 0-07-054235-X. 
• Bourbaki, Nicolas (1998), General topology. Chapters 5–10, Elements of Mathematics, Berlin, New York: Springer-Verlag, ISBN 978-3-540-64563-4, MR 1726872 .
• Dieudonné, Jean (1988), Foundations of modern analysis, Academic Press, ISBN 978-0-12-215507-9 
• Droniou, Jérôme; Eymard, Robert (2016), „Uniform-in-time convergence of numerical methods for non-linear degenerate parabolic equations”, Numer. Math., 132 (4): 721—766, S2CID 5287603, arXiv:2003.09067 , doi:10.1007/s00211-015-0733-6 .
• Dunford, Nelson; Schwartz, Jacob T. (1958), Linear operators, volume 1, Wiley-Interscience .
• Fréchet, Maurice (1906), „Sur quelques points du calcul fonctionnel”, Rend. Circ. Mat. Palermo, 22: 1—74, S2CID 123251660, doi:10.1007/BF03018603, hdl:10338.dmlcz/100655 .
• Arzelà-Ascoli theorem at Encyclopaedia of Mathematics
• Kelley, J. L. (1991), General topology, Springer-Verlag, ISBN 978-0-387-90125-1 
• Kelley, J. L.; Namioka, I. (1982), Linear Topological Spaces, Springer-Verlag, ISBN 978-0-387-90169-5 
• Rudin, Walter (1976), Principles of mathematical analysis, McGraw-Hill, ISBN 978-0-07-054235-8 
• Osgood, W. F. (1898). „Beweis der Existenz einer Lösung der Differentialgleichung dy/dx = f(x, y) ohne Hinzunahme der Cauchy-Lipschitzchen Bedingung”. Monatshefte für Mathematik. 9: 331—345. S2CID 122312261. doi:10.1007/BF01707876. 
• Coddington, Earl A.; Levinson, Norman (1955). Theory of Ordinary Differential Equations. New York: McGraw-Hill. 
• Murray, Francis J.; Miller, Kenneth S. (1976) [1954]. Existence Theorems for Ordinary Differential Equations (Reprint изд.). New York: Krieger. 
• Teschl, Gerald (2012). Ordinary Differential Equations and Dynamical Systems. Providence: American Mathematical Society. ISBN 978-0-8218-8328-0. 

Spoljašnje veze

• Countably compact at PlanetMath.org.
• Sundström, Manya Raman (2010). „A pedagogical history of compactness”. arXiv:1006.4131v1  [math.HO].