Паралелограм

Паралелограм
Овај паралелограм је ромбоид јер нема праве углове и неједнаке странице.
Тип	четвороугао
Ивице и темена	4
Симетрична група	C2, [2]+, (22)
Површина	b × h (основица × висина); ab sin θ (производ суседних страница и синус било ког угла темена)
Својства	конвексан

У Еуклидовој геометрији, паралелограм је једноставан (не-самосекући) четвороугао са два пара паралелних страница. Наспрамне странице паралелограма су једнаке дужине, а наспрамни углови су једнаке мере.

Подударност наспрамних страница и наспрамних углова је директна последица Еуклидовог постулата паралелности и ни један услов се не може доказати без примењивања Еуклидовог постулата паралелности или једне од његових еквивалентних формулација.

Поређења ради, четвороугао са само једним паром паралелних страница је трапез.

Тродимензионални еквивалент паралелограма је паралелепипед.

Етимологија (на грчком грч. παραλληλ-όγραμμον — „облик од паралелних линија”) одражава дефиницију.

Посебни случајеви

Четвороуглови по симетрији

• Ромбоид — четвороугао чије су наспрамне странице паралелне, суседне странице неједнаке и чији углови нису прави.
• Правоугаоник — паралелограм са четири угла једнаке величине.
• Ромб — паралелограм са четири страница једнаке дужине.
• Квадрат — паралелограм са четири страница једнаке дужине и углова једнаке величине (прави углови).

Карактеризација

Паралелограм је једноставан (не-самосекући) четвороугао ако и само ако је једна од следећих изјава тачна:

• два пара наспрамних страница су једнаке по дужини;
• два пара наспрамних углова су једнаки по мери;
• дијагонале се узајамно полове;
• један пар наспрамних страница је паралелан и једнак по дужини;
• суседни углови су суплементни;
• свака дијагонала дели четвороугао на два подударна троугла;
• збир квадрата страница једнак је збиру квадрата дијагонала (Ово је паралелограмски закон.);
• има ротациону симетрију реда 2;
• збир удаљености од било које унутрашње тачке до страница је независна од локације тачке. (Ово је проширење Вивианијеве теореме.)
• Постоји тачка X у равни четвороугла са својством да свака права линија кроз X дели четвороугао на два подручја једнаке површине.

Стога, сви паралелограми имају сва горенаведена својства, и обрнуто; ако је само једна од ових изјава тачна у једноставном четвороуглу, онда је то паралелограм.

Формуле

Висине	$h_{a}\,=\,b\cdot \sin \alpha =b\cdot \sin \beta$ $h_{b}\,=\,a\cdot \sin \alpha =a\cdot \sin \beta$
Дијагонале	$e={\sqrt {a^{2}+b^{2}+2\cdot a\cdot b\cdot \cos \alpha }}$ $f={\sqrt {a^{2}+b^{2}-2\cdot a\cdot b\cdot \cos \alpha }}$
Обим	$O=2(a+b)\,$
Површина	$P=ah_{a}=bh_{b}=\left|\left|\,{\overrightarrow {AB}}\,\times \,{\overrightarrow {AD}}\,\right|\right|$ $S\,=\,a\cdot b\cdot \sin \alpha =a\cdot b\cdot \sin \beta ={\frac {1}{2}}\cdot e\cdot f\cdot \sin \theta$
Закон паралелограма	$e^{2}+f^{2}=2\left(a^{2}+b^{2}\right)$

Формула површине

A parallelogram can be rearranged into a rectangle with the same area.

Animation for the area formula $K=bh$.

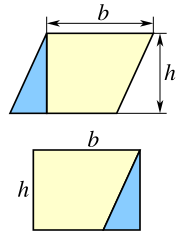
All of the area formulas for general convex quadrilaterals apply to parallelograms. Further formulas are specific to parallelograms:

A parallelogram with base b and height h can be divided into a trapezoid and a right triangle, and rearranged into a rectangle, as shown in the figure to the left. This means that the area of a parallelogram is the same as that of a rectangle with the same base and height:

$K=bh.$

The area of the parallelogram is the area of the blue region, which is the interior of the parallelogram

The base × height area formula can also be derived using the figure to the right. The area K of the parallelogram to the right (the blue area) is the total area of the rectangle less the area of the two orange triangles. The area of the rectangle is

$K_{\text{rect}}=(B+A)\times H\,$

and the area of a single orange triangle is

$K_{\text{tri}}={\frac {A}{2}}\times H.\,$

Therefore, the area of the parallelogram is

$K=K_{\text{rect}}-2\times K_{\text{tri}}=((B+A)\times H)-(A\times H)=B\times H.$

Another area formula, for two sides B and C and angle θ, is

$K=B\cdot C\cdot \sin \theta .\,$

The area of a parallelogram with sides B and C (B ≠ C) and angle $\gamma$ at the intersection of the diagonals is given by

$K={\frac {|\tan \gamma |}{2}}\cdot \left|B^{2}-C^{2}\right|.$

When the parallelogram is specified from the lengths B and C of two adjacent sides together with the length D₁ of either diagonal, then the area can be found from Heron's formula. Specifically it is

$K=2{\sqrt {S(S-B)(S-C)(S-D_{1})}}$

where $S=(B+C+D_{1})/2$ and the leading factor 2 comes from the fact that the chosen diagonal divides the parallelogram into two congruent triangles.

Area in terms of Cartesian coordinates of vertices

Let vectors $\mathbf {a} ,\mathbf {b} \in \mathbb {R} ^{2}$ and let $V={\begin{bmatrix}a_{1}&a_{2}\\b_{1}&b_{2}\end{bmatrix}}\in \mathbb {R} ^{2\times 2}$ denote the matrix with elements of a and b. Then the area of the parallelogram generated by a and b is equal to $|\det(V)|=|a_{1}b_{2}-a_{2}b_{1}|\,$.

Let vectors $\mathbf {a} ,\mathbf {b} \in \mathbb {R} ^{n}$ and let $V={\begin{bmatrix}a_{1}&a_{2}&\dots &a_{n}\\b_{1}&b_{2}&\dots &b_{n}\end{bmatrix}}\in \mathbb {R} ^{2\times n}$. Then the area of the parallelogram generated by a and b is equal to ${\sqrt {\det(VV^{\mathrm {T} })}}$.

Let points $a,b,c\in \mathbb {R} ^{2}$. Then the area of the parallelogram with vertices at a, b and c is equivalent to the absolute value of the determinant of a matrix built using a, b and c as rows with the last column padded using ones as follows:

$K=\left|\,\det {\begin{bmatrix}a_{1}&a_{2}&1\\b_{1}&b_{2}&1\\c_{1}&c_{2}&1\end{bmatrix}}\right|.$

Доказ да се дијагонале деле једна на другу

Parallelogram ABCD

To prove that the diagonals of a parallelogram bisect each other, we will use congruent triangles:

$\angle ABE\cong \angle CDE$ (alternate interior angles are equal in measure)

$\angle BAE\cong \angle DCE$ (alternate interior angles are equal in measure).

(since these are angles that a transversal makes with parallel lines AB and DC).

Also, side AB is equal in length to side DC, since opposite sides of a parallelogram are equal in length.

Therefore, triangles ABE and CDE are congruent (ASA postulate, two corresponding angles and the included side).

Therefore,

$AE=CE$

$BE=DE.$

Since the diagonals AC and BD divide each other into segments of equal length, the diagonals bisect each other.

Separately, since the diagonals AC and BD bisect each other at point E, point E is the midpoint of each diagonal.

Решетка паралелограма

Parallelograms can tile the plane by translation. If edges are equal, or angles are right, the symmetry of the lattice is higher. These represent the four Bravais lattices in 2 dimensions.

Lattices

Form	Square	Rectangle	Rhombus	Parallelogram
System	Square (tetragonal)	Rectangular (orthorhombic)	Centered rectangular (orthorhombic)	Oblique (monoclinic)
Constraints	α=90°, a=b	α=90°	a=b	None
Symmetry	p4m, [4,4], order 8n	pmm, [∞,2,∞], order 4n		p1, [∞+,2,∞+], order 2n
Form

Литература

• Abbott, Timothy Good (2008), „3.1.2 Contraparallelograms”, Generalizations of Kempe's Universality Theorem (PDF) (Master's thesis), Massachusetts Institute of Technology, стр. 34—36 
• Coxeter, H. S. M.; Longuet-Higgins, M. S.; Miller, J. C. P. (1954), „Uniform polyhedra”, Philosophical Transactions of the Royal Society of London. Series A, Mathematical and Physical Sciences, 246 (916): 401—450, Bibcode:1954RSPTA.246..401C, JSTOR 91532, MR 0062446, S2CID 202575183, doi:10.1098/rsta.1954.0003 
• Alsina, Claudi; Nelsen, Roger B. (2020), A Cornucopia of Quadrilaterals, The Dolciani Mathematical Expositions, 55, Providence, Rhode Island: MAA Press and American Mathematical Society, стр. 212, ISBN 978-1-4704-5312-1, MR 4286138 
• Cundy, H. Martyn (март 2005), „89.23 The lemniscate of Bernoulli”, The Mathematical Gazette, 89 (514): 89—93, S2CID 125521872, doi:10.1017/s0025557200176855 CS1 одржавање: Формат датума (веза)
• Begalla, Engjëll; Perucca, Antonella (2020), „The ABCD of cyclic quadrilaterals”, Uitwiskeling, University of Luxembourg, hdl:10993/43232 
• Dijksman, E. A. (1976), Motion Geometry of Mechanisms, Cambridge University Press, стр. 203, ISBN 9780521208413 
• Demaine, Erik; O'Rourke, Joseph (2007), Geometric Folding Algorithms, Cambridge University Press, стр. 32—33, ISBN 978-0-521-85757-4, MR 2354878, doi:10.1017/CBO9780511735172 
• Grebenikov, Evgenii A.; Ikhsanov, Ersain V.; Prokopenya, Alexander N. (2006), „Numeric-symbolic computations in the study of central configurations in the planar Newtonian four-body problem”, Computer algebra in scientific computing, Lecture Notes in Comput. Sci., 4194, Berlin: Springer, стр. 192—204, MR 2279793, doi:10.1007/11870814_16 
• Glaeser, Georg (2020), „Antiparallelograms; It does not always have to be a uniform rotation ...”, Geometry and its Applications in Arts, Nature and Technology, Springer International Publishing, стр. 428—429, ISBN 978-3-030-61397-6, S2CID 241160811, doi:10.1007/978-3-030-61398-3 
• De Villiers, Michael (2015), „Slaying a geometrical 'monster': finding the area of a crossed quadrilateral”, Learning and Teaching Mathematics, 2015 (18): 23—28, hdl:10520/EJC175721 
• Muirhead, R. F. (фебруар 1901), „Geometry of the isosceles trapezium and the contra-parallelogram, with applications to the geometry of the ellipse”, Proceedings of the Edinburgh Mathematical Society, 20: 70—72, doi:10.1017/s0013091500032892  CS1 одржавање: Формат датума (веза)
• Norton, Robert L. (2003), Design of Machinery, McGraw-Hill Professional, стр. 51, ISBN 978-0-07-121496-4 
• Bryant, John; Sangwin, Christopher J. (2008), „3.3 The Crossed Parallelogram”, How Round Is Your Circle? Where Engineering and Mathematics Meet, Princeton University Press, стр. 54—56, ISBN 978-0-691-13118-4 
• Sossinsky, Alexey (2016), „Configuration spaces of planar linkages”, Handbook of Teichmüller theory, Vol. VI, IRMA Lectures in Mathematics and Theoretical Physics, 27, Zürich: European Mathematical Society, стр. 335—373, MR 3618193 

• van Schooten, Frans (1646), De Organica Conicarum Sectionum In Plano Descriptione, Tractatus. Geometris, Opticis; Præsertim verò Gnomonicis et Mechanicis Utilis. Cui subnexa est Appendix, de Cubicarum Æquationum resolutione, стр. 49—50, 69—70 

• Yates, Robert C. (март 1941), „The trisection problem”, National Mathematics Magazine, 15 (6): 278—293, JSTOR 3028413, doi:10.2307/3028413 CS1 одржавање: Формат датума (веза)

Спољашње везе

• Parallelogram and Rhombus - Animated course (Construction, Circumference, Area)
• Weisstein, Eric W. „Parallelogram”. MathWorld. 
• Interactive Parallelogram --sides, angles and slope
• Area of Parallelogram at cut-the-knot
• Equilateral Triangles On Sides of a Parallelogram at cut-the-knot
• Definition and properties of a parallelogram with animated applet
• Interactive applet showing parallelogram area calculation interactive applet