Алгебарска топологија

Торус, један од најчешће проучаваних објеката у алгебарској топологији

Алгебарска топологија је грана математике која се користи алатима апстрактне алгебре у проучавању тополошких простора. Основни циљ је проналажење алгебарских инваријанти које класификују тополошке просторе до на хомеоморфизам. У многим ситуацијама је ово исувише амбициозно, па је разумније поставити скромнији циљ, класификација до на хомотопску еквиваленцију.

Мада алгебарска топологија обично користи алгебру за проучавање тополошких проблема, обратан случај, коришћење топологије за решавање алгебарских проблема, је такође понекад могућ. Алгебарска топологија на пример, омогућава згодан доказ да је свака подгрупа слободне групе такође слободна група.

Главне гране алгебарске топологије

Below are some of the main areas studied in algebraic topology:

Homotopy groups

Главни чланак: Homotopy group

In mathematics, homotopy groups are used in algebraic topology to classify topological spaces. The first and simplest homotopy group is the fundamental group, which records information about loops in a space. Intuitively, homotopy groups record information about the basic shape, or holes, of a topological space.

Homology

Главни чланак: Homology

In algebraic topology and abstract algebra, homology (in part from Greek ὁμός homos "identical") is a certain general procedure to associate a sequence of abelian groups or modules with a given mathematical object such as a topological space or a group.

Cohomology

Главни чланак: Cohomology

In homology theory and algebraic topology, cohomology is a general term for a sequence of abelian groups defined from a cochain complex. That is, cohomology is defined as the abstract study of cochains, cocycles, and coboundaries. Cohomology can be viewed as a method of assigning algebraic invariants to a topological space that has a more refined algebraic structure than does homology. Cohomology arises from the algebraic dualization of the construction of homology. In less abstract language, cochains in the fundamental sense should assign 'quantities' to the chains of homology theory.

Manifolds

Главни чланак: Manifold

A manifold is a topological space that near each point resembles Euclidean space. Examples include the plane, the sphere, and the torus, which can all be realized in three dimensions, but also the Klein bottle and real projective plane which cannot be embedded in three dimensions, but can be embedded in four dimensions. Typically, results in algebraic topology focus on global, non-differentiable aspects of manifolds; for example Poincaré duality.

Knot theory

Главни чланак: Knot theory

Knot theory is the study of mathematical knots. While inspired by knots that appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined so that it cannot be undone. In precise mathematical language, a knot is an embedding of a circle in 3-dimensional Euclidean space, $\mathbb {R} ^{3}$. Two mathematical knots are equivalent if one can be transformed into the other via a deformation of $\mathbb {R} ^{3}$ upon itself (known as an ambient isotopy); these transformations correspond to manipulations of a knotted string that do not involve cutting the string or passing the string through itself.

Complexes

Главни чланци: Simplicial complex и CW complex

A simplicial 3-complex.

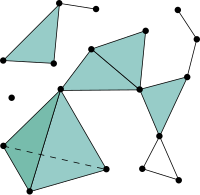
A simplicial complex is a topological space of a certain kind, constructed by "gluing together" points, line segments, triangles, and their n-dimensional counterparts (see illustration). Simplicial complexes should not be confused with the more abstract notion of a simplicial set appearing in modern simplicial homotopy theory. The purely combinatorial counterpart to a simplicial complex is an abstract simplicial complex.

A CW complex is a type of topological space introduced by J. H. C. Whitehead to meet the needs of homotopy theory. This class of spaces is broader and has some better categorical properties than simplicial complexes, but still retains a combinatorial nature that allows for computation (often with a much smaller complex).

Метода алгебарских инваријанти

An older name for the subject was combinatorial topology, implying an emphasis on how a space X was constructed from simpler ones (the modern standard tool for such construction is the CW complex). In the 1920s and 1930s, there was growing emphasis on investigating topological spaces by finding correspondences from them to algebraic groups, which led to the change of name to algebraic topology. The combinatorial topology name is still sometimes used to emphasize an algorithmic approach based on decomposition of spaces.

In the algebraic approach, one finds a correspondence between spaces and groups that respects the relation of homeomorphism (or more general homotopy) of spaces. This allows one to recast statements about topological spaces into statements about groups, which have a great deal of manageable structure, often making these statement easier to prove. Two major ways in which this can be done are through fundamental groups, or more generally homotopy theory, and through homology and cohomology groups. The fundamental groups give us basic information about the structure of a topological space, but they are often nonabelian and can be difficult to work with. The fundamental group of a (finite) simplicial complex does have a finite presentation.

Homology and cohomology groups, on the other hand, are abelian and in many important cases finitely generated. Finitely generated abelian groups are completely classified and are particularly easy to work with.

Литература

• Ayres, Frank (1965). Schaum's Outline of Modern Abstract Algebra (1st изд.). McGraw-Hill. ISBN 978-0-07-002655-1. .
• Allegretti, Dylan G. L. (2008), Simplicial Sets and van Kampen's Theorem  (Discusses generalized versions of van Kampen's theorem applied to topological spaces and simplicial sets).
• Bredon, Glen E. (1993), Topology and Geometry, Graduate Texts in Mathematics, 139, Springer, ISBN 0-387-97926-3 .
• Brown, R. (2007), Higher dimensional group theory  (Gives a broad view of higher-dimensional van Kampen theorems involving multiple groupoids).
• Brown, R.; Razak, A. (1984), „A van Kampen theorem for unions of non-connected spaces”, Arch. Math., 42: 85—88, S2CID 122228464, doi:10.1007/BF01198133 . "Gives a general theorem on the fundamental groupoid with a set of base points of a space which is the union of open sets."
• Brown, R.; Hardie, K.; Kamps, H.; Porter, T. (2002), „The homotopy double groupoid of a Hausdorff space”, Theory Appl. Categories, 10 (2): 71—93 .
• Brown, R.; Higgins, P.J. (1978), „On the connection between the second relative homotopy groups of some related spaces”, Proc. London Math. Soc., S3-36 (2): 193—212, doi:10.1112/plms/s3-36.2.193 . "The first 2-dimensional version of van Kampen's theorem."
• Brown, Ronald; Higgins, Philip J.; Sivera, Rafael (2011), Nonabelian Algebraic Topology: Filtered Spaces, Crossed Complexes, Cubical Homotopy Groupoids, European Mathematical Society Tracts in Mathematics, 15, European Mathematical Society, ISBN 978-3-03719-083-8, arXiv:math/0407275 , Архивирано из оригинала 2009-06-04. г.  This provides a homotopy theoretic approach to basic algebraic topology, without needing a basis in singular homology, or the method of simplicial approximation. It contains a lot of material on crossed modules.
• Fraleigh, John B. (1976), A First Course In Abstract Algebra (2nd изд.), Reading: Addison-Wesley, ISBN 0-201-01984-1 
• Greenberg, Marvin J.; Harper, John R. (1981), Algebraic Topology: A First Course, Revised edition, Mathematics Lecture Note Series, Westview/Perseus, ISBN 9780805335576 . A functorial, algebraic approach originally by Greenberg with geometric flavoring added by Harper.
• Hatcher, Allen (2002), Algebraic Topology, Cambridge: Cambridge University Press, ISBN 0-521-79540-0 . A modern, geometrically flavoured introduction to algebraic topology.
• Higgins, Philip J. (1971), Notes on categories and groupoids, Van Nostrand Reinhold, ISBN 9780442034061 
• Maunder, C. R. F. (1970), Algebraic Topology, London: Van Nostrand Reinhold, ISBN 0-486-69131-4 .
• tom Dieck, Tammo (2008), Algebraic Topology, EMS Textbooks in Mathematics, European Mathematical Society, ISBN 978-3-03719-048-7 
• van Kampen, Egbert (1933), „On the connection between the fundamental groups of some related spaces”, American Journal of Mathematics, 55 (1): 261—7, JSTOR 51000091 
• Hazewinkel Michiel, ур. (2001). „Algebraic topology”. Encyclopaedia of Mathematics. Springer. ISBN 978-1556080104. 
• May, J. Peter (1999). A Concise Course in Algebraic Topology (PDF). University of Chicago Press. Приступљено 2008-09-27.  Section 2.7 provides a category-theoretic presentation of the theorem as a colimit in the category of groupoids.
• Ronald Brown, `Groupoids and crossed objects in algebraic topology', Homology, Homotopy and Applications, 1 (1999) 1–78.
• Čech, Eduard (1932), „Höherdimensionale Homotopiegruppen”, Verhandlungen des Internationalen Mathematikerkongress, Zürich .
• Hazewinkel Michiel, ур. (2001). „Homotopy group”. Encyclopaedia of Mathematics. Springer. ISBN 978-1556080104. 
• Hopf, Heinz (1931), „Über die Abbildungen der dreidimensionalen Sphäre auf die Kugelfläche”, Mathematische Annalen, 104 (1): 637—665, doi:10.1007/BF01457962 .
• Kamps, Klaus H.; Porter, Timothy (1997). Abstract homotopy and simple homotopy theory. River Edge, NJ: World Scientific Publishing. ISBN 981-02-1602-5. MR 1464944. doi:10.1142/9789812831989. 
• Toda, Hiroshi (1962). Composition methods in homotopy groups of spheres. Annals of Mathematics Studies. 49. Princeton, N.J.: Princeton University Press. ISBN 0-691-09586-8. MR 0143217. 
• Whitehead, George William (1978). Elements of homotopy theory. Graduate Texts in Mathematics. 61 (3rd изд.). New York-Berlin: Springer-Verlag. стр. xxi+744. ISBN 978-0-387-90336-1. MR 0516508. 
• van den Berg, J.B.; Ghrist, R.; Vandervorst, R.C.; Wójcik, W. (2015). „Braid Floer homology” (PDF). Journal of Differential Equations. 259 (5): 1663—1721. Bibcode:2015JDE...259.1663V. S2CID 16865053. doi:10.1016/j.jde.2015.03.022 . 
• Pellikka, M; S. Suuriniemi; L. Kettunen; C. Geuzaine (2013). „Homology and Cohomology Computation in Finite Element Modeling” (PDF). SIAM J. Sci. Comput. 35 (5): B1195—B1214. CiteSeerX 10.1.1.716.3210 . doi:10.1137/130906556. 
• Arnold, Douglas N.; Richard S. Falk; Ragnar Winther (16. 5. 2006). „Finite element exterior calculus, homological techniques, and applications”. Acta Numerica. 15: 1—155. Bibcode:2006AcNum..15....1A. S2CID 122763537. doi:10.1017/S0962492906210018. CS1 одржавање: Формат датума (веза)
• Cartan, Henri Paul; Eilenberg, Samuel (1956). Homological Algebra. Princeton mathematical series. 19. Princeton University Press. ISBN 9780674079779. OCLC 529171. 
• Eilenberg, Samuel; Moore, J.C. (1965). Foundations of relative homological algebra. Memoirs of the American Mathematical Society number. 55. American Mathematical Society. ISBN 9780821812556. OCLC 1361982. 
• Gowers, Timothy; Barrow-Green, June; Leader, Imre, ур. (2010), The Princeton Companion to Mathematics, Princeton University Press, ISBN 9781400830398 .
• Hatcher, A. (2002), Algebraic Topology, Cambridge University Press, ISBN 0-521-79540-0 . Detailed discussion of homology theories for simplicial complexes and manifolds, singular homology, etc.
• Hilton, Peter (1988), „A Brief, Subjective History of Homology and Homotopy Theory in This Century”, Mathematics Magazine, Mathematical Association of America, 60 (5): 282—291, JSTOR 2689545, doi:10.1080/0025570X.1988.11977391 
• Richeson, D. (2008), Euler's Gem: The Polyhedron Formula and the Birth of Topology, Princeton University .
• Spanier, Edwin H. (1966), Algebraic Topology, Springer, стр. 155, ISBN 0-387-90646-0 .
• Stillwell, John (1993), Classical Topology and Combinatorial Group Theory, Springer, ISBN 978-0-387-97970-0, doi:10.1007/978-1-4612-4372-4_6 .
• Teicher, M., ур. (1999), The Heritage of Emmy Noether, Israel Mathematical Conference Proceedings, Bar-Ilan University/American Mathematical Society/Oxford University Press, ISBN 978-0-19-851045-1, OCLC 223099225 
• Weibel, Charles A. (1999), „28. History of Homological Algebra” (PDF), Ур.: James, I. M., History of Topology, Elsevier, ISBN 9780080534077 .
• Dieudonné, Jean (1989), History of Algebraic and Differential Topology, Birkhäuser, ISBN 0-8176-3388-X, MR 0995842 
• Dold, Albrecht (1972), Lectures on Algebraic Topology, Springer-Verlag, ISBN 978-3-540-58660-9, MR 0415602 
• Eilenberg, Samuel; Steenrod, Norman (1952), Foundations of Algebraic Topology, Princeton University Press, ISBN 9780691627236, MR 0050886 
• Hartshorne, Robin (1977), Algebraic Geometry, Graduate Texts in Mathematics, 52, New York, Heidelberg: Springer-Verlag, ISBN 0-387-90244-9, MR 0463157 
• Hatcher, Allen (2001), Algebraic Topology, Cambridge University Press, ISBN 0-521-79540-0, MR 1867354 
• Hazewinkel Michiel, ур. (2001). „Cohomology”. Encyclopaedia of Mathematics. Springer. ISBN 978-1556080104. .
• May, J. Peter (1999), A Concise Course in Algebraic Topology (PDF), University of Chicago Press, ISBN 0-226-51182-0, MR 1702278 
• Switzer, Robert (1975), Algebraic Topology — Homology and Homotopy, Springer-Verlag, ISBN 3-540-42750-3, MR 0385836 
• Thom, René (1954), „Quelques propriétés globales des variétés différentiables”, Commentarii Mathematici Helvetici, 28: 17—86, MR 0061823, doi:10.1007/BF02566923 

Спољашње везе

• [1] N.J. Windberger intro to algebraic topology, last six lectures with an easy intro to homology
• [2] Algebraic topology Allen Hatcher - Chapter 2 on homology
• Homology group at Encyclopaedia of Mathematics